Chapter 13: Direct and Inverse Proportions

 

Introduction to Proportions

Direct and Inverse Proportions ,In mathematics, a proportion describes a relationship between two quantities. When two quantities change in such a way that their ratio remains constant or their product remains constant, they are said to be in proportion. Chapter 13 of the 8th-grade mathematics curriculum focuses on two types of proportions: direct proportion and inverse proportion. These concepts are essential for understanding real-world relationships, such as speed and time, cost and quantity, or work and time. This chapter introduces students to the foundational concepts, problem-solving techniques, and applications of proportions.

---

1. Direct Proportion

Definition

Two quantities are said to be in direct proportion if an increase in one quantity causes a proportional increase in the other, or a decrease in one causes a proportional decrease in the other. Mathematically, if two quantities ( x ) and ( y ) are in direct proportion, their ratio remains constant: [ \frac{x}{y} = k \quad \text{or} \quad x = k \cdot y ] Here, ( k ) is the constant of proportionality.

Characteristics

• When one quantity doubles, the other also doubles.

• When one quantity is halved, the other is halved.

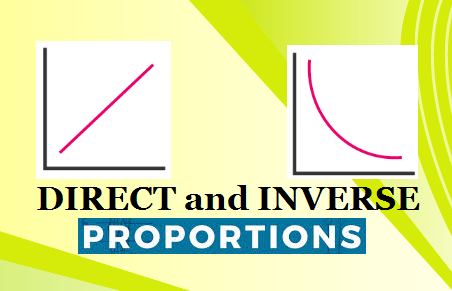
• The graph of two quantities in direct proportion is a straight line passing through the origin (0, 0).

Examples

1. Cost and Quantity: If 1 kg of apples costs $2, then 2 kg costs $4, 3 kg costs $6, and so on. The cost is directly proportional to the quantity.

2. Distance and Time: If a car travels at a constant speed, the distance covered is directly proportional to the time taken.

Formula

For two quantities ( x ) and ( y ) in direct proportion: [ \frac{x_1}{y_1} = \frac{x_2}{y_2} ] This is known as the proportion equation, where ( (x_1, y_1) ) and ( (x_2, y_2) ) are corresponding pairs of values.

Solving Problems

To solve problems involving direct proportion:

1. Identify the two quantities in proportion.

2. Set up the proportion equation using given values.

3. Solve for the unknown by cross-multiplying.

Example Problem: If 5 pens cost $20, how much will 8 pens cost?

• Let the cost of 8 pens be ( x ).

• Since the cost is directly proportional to the number of pens: [ \frac{5}{20} = \frac{8}{x} ]

• Cross-multiply: [ 5x = 20 \cdot 8 \implies 5x = 160 \implies x = 32 ]

• Therefore, 8 pens cost $32.

---

2. Inverse Proportion

Definition

Two quantities are said to be in inverse proportion if an increase in one quantity causes a proportional decrease in the other, and vice versa. Mathematically, if two quantities ( x ) and ( y ) are in inverse proportion, their product remains constant: [ x \cdot y = k ] Here, ( k ) is a constant.

Characteristics

• When one quantity doubles, the other is halved.

• When one quantity is halved, the other doubles.

• The graph of two quantities in inverse proportion is a hyperbola.

Examples

1. Speed and Time: If a car covers a fixed distance, the time taken is inversely proportional to the speed. Higher speed means less time.

2. Workers and Time: If more workers are employed to complete a task, the time taken decreases.

Formula

For two quantities ( x ) and ( y ) in inverse proportion: [ x_1 \cdot y_1 = x_2 \cdot y_2 ] This equation relates the pairs of values ( (x_1, y_1) ) and ( (x_2, y_2) ).

Solving Problems

To solve problems involving inverse proportion:

1. Identify the two quantities in inverse proportion.

2. Set up the equation using the product rule.

3. Solve for the unknown.

Example Problem: If 6 workers can complete a job in 12 days, how many days will it take for 8 workers to complete the same job?

• Let the number of days for 8 workers be ( y ).

• Since the number of workers and days are inversely proportional: [ 6 \cdot 12 = 8 \cdot y ]

• Simplify: [ 72 = 8y \implies y = \frac{72}{8} = 9 ]

• Therefore, 8 workers will take 9 days.

---

3. Differences Between Direct and Inverse Proportions

Aspect	Direct Proportion	Inverse Proportion
Relationship	Increase in one increases the other.	Increase in one decreases the other.
Mathematical Form	( \frac{x}{y} = k ) or ( x = k \cdot y )	( x \cdot y = k )
Graph	Straight line through origin.	Hyperbola.
Example	Cost and quantity.	Speed and time.

---

4. Applications in Real Life

Proportions are widely applicable in everyday scenarios:

• Direct Proportion:

  • Shopping: Calculating the cost of groceries based on quantity.

  • Fuel Consumption: Fuel used is proportional to the distance traveled.

  • Scaling Recipes: Adjusting ingredient quantities for more servings.

• Inverse Proportion:

  • Travel Planning: Estimating travel time based on speed.

  • Workforce Management: Determining the number of workers needed to meet a deadline.

  • Resource Allocation: Dividing resources among a varying number of people.

---

5. Problem-Solving Strategies

For Direct Proportion

1. Determine the constant of proportionality using known values.

2. Use the proportion equation to find the unknown.

3. Verify the solution by checking the ratio.

For Inverse Proportion

1. Use the product rule to set up the equation.

2. Solve for the unknown variable.

3. Check if the product of corresponding pairs is constant.

Tips

• Read the problem carefully to identify whether it involves direct or inverse proportion.

• Use units to ensure consistency (e.g., cost in dollars, time in hours).

• Practice converting word problems into mathematical equations.

---

6. Common Mistakes to Avoid

1. Confusing Direct and Inverse Proportions:

   • If one quantity increases and the other decreases, it’s inverse proportion.

   • If both increase or decrease together, it’s direct proportion.

2. Incorrect Setup of Equations:

   • For direct proportion, ensure ratios are equal.

   • For inverse proportion, ensure products are equal.

3. Unit Errors:

   • Always check that units match when setting up proportions.

4. Calculation Errors:

   • Double-check cross-multiplication or division steps.

---

7. Practice Questions

1. Direct Proportion: A car travels 150 km in 3 hours. How far will it travel in 5 hours at the same speed?

2. Inverse Proportion: If 10 pipes can fill a tank in 12 minutes, how long will it take for 15 pipes to fill the same tank?

3. Mixed Problem: If 4 books cost $24, how much will 7 books cost? Is this direct or inverse proportion?

---

8. Summary

• Direct Proportion: Two quantities increase or decrease together, maintaining a constant ratio (( \frac{x}{y} = k )).

• Inverse Proportion: One quantity increases as the other decreases, maintaining a constant product (( x \cdot y = k )).

• Key Skills: Setting up proportion equations, solving for unknowns, and applying concepts to real-world problems.

• Importance: Understanding proportions helps in problem-solving across mathematics, science, and daily life.

Download pdf notes and Sulutions of the Chapter:

 

Chapter 14: Factorisation

 

Please Visit Readspot for Hindi Medium Study Material