Symmetry – Class 6 Mathematics Notes

Introduction to Symmetry

Symmetry is an important concept in mathematics, art, and nature. It is a way of describing balance and harmony in shapes and objects. Symmetry is when an object or figure can be divided into identical parts that mirror each other. Symmetry helps us understand the beauty of shapes and patterns in the world around us.

In Class 6 Mathematics, symmetry focuses on understanding what symmetry is, identifying symmetric objects, and learning how to draw symmetric figures.

What is Symmetry?

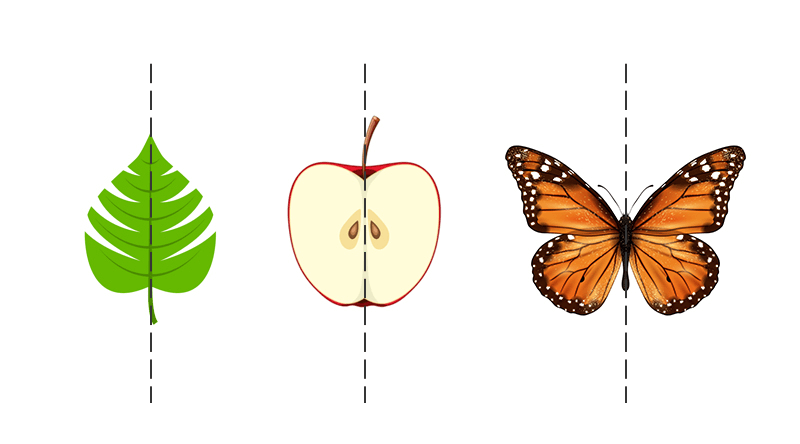
A shape or figure is said to be symmetric if it can be divided into two or more identical parts that are mirror images of each other. The dividing line along which the figure is folded to get identical parts is called the line of symmetry.

For example:

• A butterfly has symmetry because its left and right wings are mirror images of each other.
• A square has symmetry because it can be divided into equal parts in several ways.

Key Terms:

1. Symmetric Figure: A figure that can be divided into two identical halves.
2. Line of Symmetry: The imaginary line that divides a figure into two identical parts.
3. Mirror Image: When one half of a figure looks like a reflection of the other half.

Types of Symmetry

Symmetry can be of different types depending on how the figure can be divided or rotated. The types of symmetry are:

1. Line Symmetry (Reflection Symmetry)

Line symmetry occurs when a figure can be divided into two identical halves along a straight line. This line is called the line of symmetry or axis of symmetry.

• The line of symmetry acts like a mirror, reflecting one half of the figure onto the other.
• A figure can have one, two, or multiple lines of symmetry.

Examples of Line Symmetry:

• A square has 4 lines of symmetry.
• An equilateral triangle has 3 lines of symmetry.
• A rectangle has 2 lines of symmetry.
• A circle has infinite lines of symmetry because it can be divided into identical parts in countless ways.

Real-life Examples:

• The wings of a butterfly.
• The leaves of a plant.
• Human faces (approximately symmetric).
• Letters such as A, H, M, O, and X in the English alphabet have line symmetry.

2. Rotational Symmetry

Rotational symmetry occurs when a figure looks the same after being rotated around a central point by a certain angle. The number of times the figure matches itself in one complete rotation (360 degrees) is called the order of rotational symmetry.

Examples of Rotational Symmetry:

• A square has rotational symmetry of order 4 (it looks the same after being rotated by 90°, 180°, 270°, and 360°).
• An equilateral triangle has rotational symmetry of order 3.
• A circle has infinite rotational symmetry.

Real-life Examples:

• A fan with three blades.
• The wheel of a car.
• The petals of some flowers.

3. Point Symmetry

Point symmetry (or central symmetry) occurs when every part of a figure has a matching part at an equal distance but in the opposite direction from a central point.

Examples of Point Symmetry:

• The letter “S” has point symmetry.
• Some star shapes have point symmetry.

Identifying Symmetry in Figures

To identify symmetry in figures, follow these steps:

1. Check for Line Symmetry: Draw a line through the figure and see if the two halves match exactly.
2. Check for Rotational Symmetry: Rotate the figure by different angles (e.g., 90°, 180°) and check if it looks the same.
3. Use Mirrors: Place a mirror along the line of symmetry to verify if one half reflects the other.

Figures with Symmetry:

• Square: 4 lines of symmetry and rotational symmetry of order 4.
• Rectangle: 2 lines of symmetry and rotational symmetry of order 2.
• Circle: Infinite lines of symmetry and rotational symmetry of infinite order.
• Equilateral Triangle: 3 lines of symmetry and rotational symmetry of order 3.

Figures Without Symmetry:

Some figures do not have symmetry. For example:

• A scalene triangle (no equal sides or angles).
• Irregular shapes like a tree branch.

Symmetry in the English Alphabet

Many letters of the English alphabet have symmetry. Let us explore:

• Line Symmetry:
  • Vertical Symmetry: A, H, I, M, O, T, U, V, W, X, Y.
  • Horizontal Symmetry: B, C, D, E, K.
• Point Symmetry: N, S, Z.

Some letters, such as F, G, P, and Q, do not have symmetry.

Symmetry in Real Life

Symmetry is found everywhere in the real world. Some common examples include:

1. Nature:
   • Butterfly wings.
   • Leaves and flowers.
   • Snowflakes (highly symmetric).
2. Human Body:
   • The human face is approximately symmetric.
   • The left and right sides of the body are almost mirror images.
3. Art and Architecture:
   • Patterns on buildings.
   • Symmetric designs in tiles, windows, and doors.
   • Monuments like the Taj Mahal have symmetric structures.
4. Everyday Objects:
   • Car wheels.
   • Playing cards.
   • Logos and symbols.

How to Draw Symmetric Figures

To draw symmetric figures:

1. Draw the line of symmetry.
2. Use a ruler and compass to measure and draw the other half of the figure.
3. Check that both halves are mirror images of each other.
4. For rotational symmetry, rotate the figure and ensure it matches itself at different angles.

Example: To draw a symmetric butterfly:

• Draw a vertical line as the line of symmetry.
• Draw half of the butterfly on one side of the line.
• Reflect the other half along the line.

Importance of Symmetry

Symmetry is important for several reasons:

1. In Mathematics: Symmetry helps us solve problems related to geometry, patterns, and designs.
2. In Art: Artists use symmetry to create beautiful and balanced designs.
3. In Nature: Symmetry is a sign of harmony and balance in natural objects.
4. In Science: Symmetry is used in physics, chemistry, and biology to study molecules, structures, and organisms.
5. In Daily Life: Symmetry is used in designing objects, architecture, and decorations.

Summary

1. Symmetry is when a figure can be divided into identical parts that mirror each other.
2. Line of Symmetry is the line that divides a figure into two mirror-image halves.
3. Types of Symmetry:
   • Line Symmetry: Figures like squares and triangles have line symmetry.
   • Rotational Symmetry: Figures that look the same when rotated, such as circles.
   • Point Symmetry: Figures with matching parts around a central point.
4. Symmetry is seen in nature, art, architecture, and everyday objects.
5. Letters like A, H, M, and O have symmetry, while irregular shapes do not.

Understanding symmetry helps us appreciate the beauty and balance in the world around us. It is an essential concept in geometry that builds a foundation for more advanced studies.

Practice Questions:

1. Draw a line of symmetry for the following shapes:
   • Square
   • Rectangle
   • Equilateral triangle
2. Identify whether the following letters have symmetry: B, C, H, M, N, S.
3. Find examples of symmetry in your home or surroundings.
4. Draw a figure with rotational symmetry of order 4.
5. Explain the importance of symmetry in real life with examples.

By understanding symmetry, students can recognize patterns, improve their drawing skills, and explore its applications in various fields.

 

Download PDF Notes and Excercise solution of the Chapter.

 

Chapter-14: Practical Geometry

Please Visit Readspot for Hindi Medium Study Material