Chapter 3: Playing with Numbers

Playing with Numbers

Playing with Numbers , is a topic in math that involves activities and exercises to help students learn about numbers and their properties. Some of the topics covered in “Playing with Numbers” include:

Factor

A factor of a number is an exact divisor of that number

Example

1. Factor of 6

1 → Since 1 exactly divides 6

2 → Since it exactly divides 6

3 → Since it exactly divides 6

6 → Since it exactly divides 6

Properties of factors:Playing with Numbers

• 1 is a factor of every number
• Every number is a factor of itself.
• Every factor of a number is an exact divisor of that number
• Every factor is less than or equal to the given number
• Number of factors of a given number are finite.

Multiple

Multiple of a number is the numbers obtained by multiplying that numbers with various Natural numbers

Example

Number is 6

Multiple will be

6 × 1 = 6

6 × 2 = 12

6 × 3 = 18

Properties of Multiple: Playing with Numbers

• Every multiple of a number is greater than or equal to that number.
• Number of multiples of a given number is infinite.
• Every number is a multiple of itself.

Perfect Number

A number for which sum of all its factors is equal to twice the number is called a perfect number

Example

1. 6

The factors of 6 are 1, 2, 3 and 6.

Now, 1 + 2 + 3 + 6 = 12 = 2 × 6

2. 28

All the factors of 28 are 1, 2, 4, 7, 14 and 28.

Now, 1 + 2 + 4 + 7 + 14 + 28 = 56 = 2 × 28

Prime Numbers: Playing with Numbers

The numbers other than 1 whose only factors are 1 and the number itself are called Prime numbers.

Example:

2, 3, 5, 7, 11 ,13

We can find list of prime numbers till 100 using Sieve of Eratosthenes method

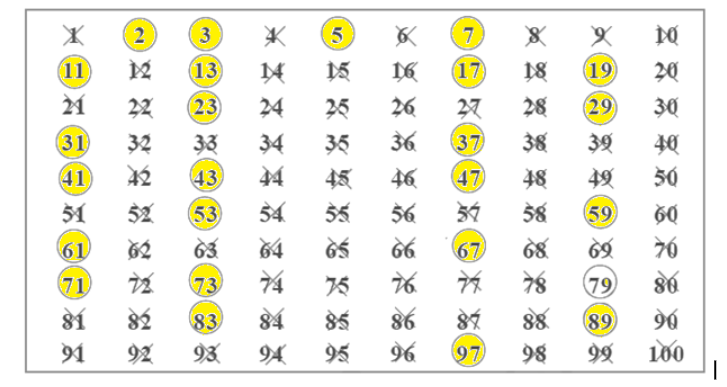
• Step 1: Cross out 1 because it is not a prime number.
• Step 2: Encircle 2, cross out all the multiples of 2, other than 2 itself, i.e. 4, 6, 8 and so on.
• Step 3: You will find that the next uncrossed number is 3. Encircle 3 and cross out all the multiples of 3, other than 3 itself.
• Step 4: The next uncrossed number is 5. Encircle 5 and cross out all the multiples of 5 other than 5 itself.
• Step 5: Continue this process till all the numbers in the list are either encircled or crossed out.

All the encircled numbers are prime numbers. All the crossed-out numbers, other than 1 are composite numbers

Composite Numbers: Playing with Numbers

Numbers having more than two factors (1 and itself) are called Composite numbers

Example:

4, 6, 8 ,9….

Zero (0) is considered as neither prime nor a composite number because it does not have any factors.

Composite Numbers Examples

The examples of composite numbers are 6, 14, 25, 30, 52, etc, such that:

Composite numbers	Factors
6	1, 2, 3, 6
14	1, 2, 7, 14
25	1, 5, 25
30	1, 2, 3, 5, 6, 10, 15, 30
52	1, 2, 4, 13, 26, 52

 

Properties of Composite Numbers:Playing with Numbers

The properties of composite numbers are easy to remember.

• Composite numbers have more than two factors
• Composite numbers are evenly divisible by their factors
• Each composite number is a factor of itself
• The smallest composite number is 4
• Each composite number will include at least two prime numbers as its factors (E.g., 10 = 2 x 5, where 2 and 5 are prime numbers)
• Composite numbers are divisible by other composite numbers also

Even Numbers:Playing with Numbers

The numbers which are multiple of 2 are called even numbers

Example

2, 4, 6, 8, 10, 12, 14

Even numbers have 0, 2, 4, 6, 8 in it one’s place

Odd Numbers:Playing with Numbers

The numbers which are not multiple of 2 are called odd numbers

Example

1,3, 5,7,9,11……

Important points about prime numbers based on definition of odd and even numbers

• 2 is the smallest prime number which is even.
• every prime number except 2 is odd.

Tests for Divisibility of Numbers:Playing with Numbers

Number	Test of divisibility
2	A number is divisible by 2 if it has any of the digits 0, 2, 4, 6 or 8 in its one’s place
3	A number is divisible by 3 if the sum of the digits is a multiple of 3, then the number is divisible by 3.   Example   153 – Sum of digit = 1+5+3=9   and 9 /3 =3 So, 153 is divisible by 3
4	1) For one and two-digit number, just check the divisibility by actual division   2) For number with 3 or more digits is divisible by 4 if the number formed by its last two digits (i.e. ones and tens) is divisible by 4.
5	A number is divisible by 5 if a number which has either 0 or 5 in its one’s place
6	A number is divisible by 6 if a number is divisible by 2 and 3 both
7	It must be checked by actual division
8	1) For one, two-digit number, three-digit and four-digit number, just check the divisibility by actual division 2) For a number with 4 or more digits is divisible by 8, if the number formed by the last three digits is divisible by 8
9	A number is divisible by 9, if the sum of the digits of a number is divisible by 9
10	A number is divisible by 10 if a number has 0 in the ones
11	A number is divisible by 11 if the difference between the sum of the digits at odd places (from the right) and the sum of the digits at even places (from the right) of the number is either 0 or divisible by 11,

 

Common Factors and Multiple:Playing with Numbers

We already studied the factors and Multiple in previous section.  We can find common factors and multiple between 2 or more numbers

Example

Common factors of 4, 12, and 16

Factors of 4 are 1, 2 and 4.

Factors of 12 are 1, 2, 3, 4, 6 and 12.

Factors of 16 are 1, 2, 4, 8 and 16.

Clearly, 1, 2 and 4 are the common factors of 4, 12, and 16.

Important Note:Playing with Numbers

Two numbers having only 1 as a common factor are called co-prime number

Examples 3 and 4 are co-prime

Divisibility Rules based on factors and Multiple, co -prime

• If a number is divisible by another number then it is divisible by each of the factors of that number

Example

36, is divisible by 18

Now if we find factors of 18 i.e., 1, 2, 3, 4, 9, 18

So, 36 is also divisible by 1, 2, 3, 4, 9, 18

• If a number is divisible by two co-prime numbers, then it is divisible by their product also

Example

45

It is divisible by 3 (4 + 5 = 9)

It is divisible by 5

Since 3, 5 are coprime. Now the product is 3 × 5 = 15. Now it is divisible by 15 also

• If two given numbers are divisible by a number, then their sum is also divisible by that number.

Example

15 is divisible by 3

 9 is divisible by 3

Sum = 15 + 9 = 24 

We can see that it is also divisible by 3

• If two given numbers are divisible by a number, then their difference is also divisible by that number

Example

15 is divisible by 3

9 is divisible by 3

Difference = 15 – 9 = 6 

We can see that it is also divisible by 3

Factorization and Prime -Factorization:Playing with Numbers

Factorization is expressing the number as a product of its factors

So,

 36 = 3 × 12 = 4 × 9

This form is called Factorization

Prime Factorization is expressing the number as a product of its prime factors

36 = 2 × 2 × 3 × 3

We can find prime factorization by dividing the numbers with 2, 3, 5, 7 etc. in this order repeatedly so long as the quotient is divisible by that number

HCF and LCM:Playing with Numbers

• The Highest Common Factor (HCF) of two or more given numbers is the highest of their common factors. It is also known as Greatest Common Divisor (GCD).

Steps to find HCF or GCD

• Find the prime factorization of the numbers
• Choose the common factors in them
• Multiply those common factors to obtain HCF

• The Lowest Common Multiple (LCM) of two or more given numbers is the lowest of their common multiples.

Steps to find LCM

• Find the prime factorization of the numbers
• Look for the maximum occurrence of all the prime factors in these numbers
• The LCM of the numbers will be the product of the prime factors counted the maximum number of times they occur in any of the numbers.

LCM using division method: Playing with Numbers

Here we divide the given numbers by common prime number until the remainder is a prime number or one. LCM will be the product obtained by multiplying all divisors and remaining prime numbers.

Steps are

• We place number in the line
• We start dividing the number by least prime number which is common among all of them or group of them
• Keep dividing by least until we have 1’s in the remainder
• LCM is the product of the divisors

Example

• Find the LCM of 14 and 20

LCM = 2 × 2 × 7 × 5 = 140

• Find the LCM of 12 ,18 and 44.

LCM = 2 × 2 × 3 × 3 × 11 = 396

Perfect numbers

A number for which sum of all its factors is equal to twice the number is called a perfect number.

Example: Factors of 28 are 1, 2, 4, 7, 14 and 28.

Here, 1 + 2 + 4 + 7 + 14 + 28 = 56 = 2 × 28

Therefore, sum of factors of 28 is equal to twice the number 28.

To know more about Perfect Numbers,

 

Important Questions of Playing with Numbers

Multiple Choice Questions:Playing with Numbers

• What are the numbers which have more than two factors called?

1. Even numbers
2. Prime numbers
3. Composite numbers
4. Odd numbers

1. The sum of two odd and one even numbers is:

1. Even
2. Prime
3. Composite
4. Odd

1. The number which is divisible by 5 is:

1. 422
2. 423
3. 424
4. 425

1. The HCF of 12 and 40 is:

1. 3
2. 4
3. 5
4. 6

1. Every ______ of a number is greater than or equal to that number.

1. factor
2. number
3. multiple
4. none to these

1. What is the H.C.F. of two co-prime numbers?

1. 1
2. 0
3. 2
4. 4

1. The LCM of 12, 15, 45 is:

1. 170
2. 180
3. 190
4. 200

1. Find the HCF of 27, 63.

1. 2
2. 6
3. 3
4. 9

1. ______ is the smallest prime number which is even:

1. 2
2. 4
3. 3
4. 1

1. If a number is divisible by 10, then which of the following can be its one’s digit?

1. 2
2. 3
3. 4
4. 0

1. The value of 10 + 40 ÷ 8 × 2 – 9 is:

1. 9
2. 10
3. 11
4. 12

1. The number which is divisible by 3 is:

1. 135
2. 136
3. 137
4. 139

1. The only prime number which is also even is

1. 6
2. 1
3. 2
4. 4

1. Which of the following is the L.C.M. of 36 and 72?

1. 36
2. 72
3. 108
4. 2

1. A prime number is a number which:

1. is divisible by 2
2. is not divisible by 2
3. has no factors
4. has exactly two factors

Match The Following:

	Column I		Column II
1.	15	A.	Factor of 50
2.	12	B.	Factor of 30
3.	25	C.	Factor of 65
4.	13	D.	Factor of 48

Fill in the blanks:Playing with Numbers

1. 1 is neither _____ nor _____.
2. The smallest prime number is _____.
3. The smallest composite number is _____.
4. The smallest even number is _____.

True /False:Playing with Numbers

1. A number with 4 or more digits is divisible by 8, if the number formed by the last three digits is divisible by 8.
2. If the sum of the digits of a number is divisible by 3, then the number itself is divisible by 9.
3. All numbers which are divisible by 4 may not be divisible by 8.
4. The Highest Common Factor of two or more numbers is greater than their Lowest Common Multiple.

 

Very Short Questions:Playing with Numbers

1. Find LCM of 60 and 40.
2. Write all the factors of the following number: 27
3. List all the multiples of 7 that lie between 125 and 142.
4. Find the H.C.F of the following numbers. 70, 105, 175
5. Find the H.C.F of the following numbers. 27, 63
6. Find all the multiples of 9 upto 100.
7. Find the least 4-digit number which is exactly divisible by 8, 10, and 12.
8. What is the sum of any two?

(a) even numbers

(b) odd numbers

1. Which of the following numbers is divisible by 3?

(a) 1212

(b) 625

1. If the LCM and HCF of any two numbers are 15 and 4 respectively, find the product of the numbers.
2. Find the HCF of 5 and 7.
3. Write first 3 multiples of 25.
4. What are the possible factors of (a) 12 (b) 18?
5. Write first three multiples of 11.
6. Write pairs of twin prime numbers less than 20.

Short Questions:Playing with Numbers

1. Simplify: 32 + 96 ÷ (7 + 9)
2. Simplify: 18 + {1 + (5 – 3) x 5}
3. Without actual division, show that 11 is a factor of 1,10,011.
4. The sum of two numbers is 25 and their product is 144. Find the numbers.
5. Is 80136 divisible by 11?
6. The HCF and LCM of two numbers are 6 and 120 respectively. If one of the numbers is 24, find the other number.
7. Find the LCM of 12 and 30.
8. Find the smallest 4-digit number which is divisible by 18, 24 and 32.
9. Find the greatest number which divides 82 and 132 leaving 1 and 6, respectively as remainders.
10. Find the greatest number that will divide 455, 582 and 710 leaving remainders 14, 15 and 17 respectively.

Long Questions:

1. Three sets of English, Hindi, and Urdu books are to be stacked in such a way that the books are stored subject wise and the height of each stack is the same. The numbers of English, Hindi and Urdu books are 336, 192 and 144 respectively. Assuring that the books have that same thickness, determine the number of stacks of English, Hindi and Urdu books.
2. Ill cows, 185 sheep and 296 goats are to be taken across a river. There is only one boat and the boatsman says; he will take the same number and same kind of animals in each trip. Find the largest number of animals in each trip and the number of trips he will have to make.
3. In a seminar, the number of participants in Mathematics, Physics and Chemistry are 60, 96 and 144 respectively. Find the number of rooms required if in each room, the same number of ‘ participants are to be seated and all of them are to be in the same subject.

Assertion and Reason Questions:Playing with Numbers

1.) Assertion (A) – The factors of 34 are 1, 2, 17 and 34 itself

Reason (R) – every factor is less than or equal to the given number.

1. a) Both A and R are true and R is the correct explanation of A
2. b) Both A and R are true but R is not the correct explanation of A
3. c) A is true but R is false
4. d) A is false but R is true

2.) Assertion (A) –The factors of 8 are 1, 2, 4, 8.

Reason (R) – every factor of a number is an exact divisor of that number.

1. a) Both A and R are true and R is the correct explanation of A
2. b) Both A and R are true but R is not the correct explanation of A
3. c) A is true but R is false
4. d) A is false but R is true

ANSWER KEY –

Multiple Choice questions:Playing with Numbers

1. C. Composite numbers
2. A. Even
3. D. 425

Explanation: 425, a number is divisible by 5 if its unit’s digit is either 0 or 5.

1. B. 4

Explanation: 4,

2 × 2 × 3

HCF = 2 × 2 = 4

40 = 2 × 2 × 2 × 5

1. C. multiple
2. A. 1
3. B. 180

Explanation: 180

LCM = 2 × 2 × 3 × 3 × 5 = 180

1. D. 9
2. A. 2
3. D. 0
4. C. 11

Explanation: 10 + 40 ÷ 8 × 2 – 9 = 10 + 5 × 2 – 9 = 10 + 10 – 9 = 20 – 9 = 11 (BODMAS Rule)

1. A. 135

Explanation: 135, 1 + 3 + 5 = 9 which is divisible by 3 therefore no. is divisible

1. C. 2
2. B. 72
3. D. has exactly two factors

Explanation: Exactly two factors are 1 and the number itself.

Match The Following:

	Column I		Column II
1.	15	B.	Factor of 30
2.	12	D.	Factor of 48
3.	25	A.	Factor of 50
4.	13	C.	Factor of 65

Fill in the blanks:

1. 1 is neither prime number nor composite number.
2. The smallest prime number is 2.
3. The smallest composite number is 4.
4. The smallest even number is 2.

True /False:

1. True
2. False
3. True
4. False

Very Short Answer:

LCM = 23 × 3 × 5 = 120

1. 27 = 1 × 27

27 = 3 × 9

Thus, all the factors of 27 are 1, 3, 9 and 27.

1. By checking all the numbers from 125 to 142 which are divisible by 7, we get 126, 133, 140.

So, the multiples of 7 that lie between 125 to 142 are 126, 133 and 140.

1. Factors of 70 are 1, 2, 5, 7, 10, 14, 35 and 70.

Factors of 105 are 1, 3, 5, 7, 15, 21, 35 and 105.

Factors of 175 are 1, 5, 7, 25, 35 and 175.

∴ Common factors of 70, 105 and 175 are 1,5,7 and 35.

Highest of these common factors is 35.

∴ H.C.F of 70, 105 and 175 is 35.

1. Factors of 27 are 1, 3, 9 and 27.

Factors of 63 are 1, 3, 7, 9, 21 and 63.

∴ Common factors of 27 and 63 are 1, 3 and 9.

Highest of these common factors is 9.

∴ H.C.F. of 27 and 63 is 9.

1. The multiples of 9 are:

9 × 1 = 9, 9 × 2 = 18, 9 × 3 = 27, 9 × 4 = 36, 9 × 5 = 45, 9 × 6 = 54, 9 × 7 = 63, 9 × 8 = 72, 9 × 9 = 81, 9 × 10 = 90, 9 × 11 = 99, 9 × 12 = 108

Since 108 is greater than 100 therefore all the multiples of 9 upto 100 are 9, 18, 27, 36, 45, 54, 63, 72, 81, 90 and 99.

1. The smallest 4-digit number is 1000.

The least number which is exactly divisible by 8, 10, and 12 will be their L.C.M.

L.C.M. of 8, 10, and 12:

L.C.M. of 8, 10, 12 = 2 × 2 × 2 × 5 × 3 = 120.

By dividing smallest 4-digit number 1000 by 120,

We find that 1000 is not exactly divisible by 120.

If we subtract 40 from 1000, we get 1000 – 40 = 960, which will be divisible by 120. But 960 is a 3-digit number. The next number to 960 which is divisible by 120 is 960 + 120 = 1080.

Therefore, the required 4-digit number divisible by 8, 10, and 12 is 1080.

1. (a) The sum of any two even numbers is even.

Example: 4 (even) + 6 (even) = 10 (even)

(b) The sum of any two odd numbers is even.

Example: 5 (odd) + 7 (odd) = 12 (even)

1. (a) Given number = 1212

Sum of the digits = 1 + 2 + 1 + 2 = 6, which is divisible by 3.

Hence, 1212 is also divisible by 3.

(b) Given number = 625

Sum of the digits = 6 + 2 + 5 = 13, which is not divisible by 3.

Hence, 625 is not divisible by 3.

1. We know that the product of the number = LCM × HCF = 15 × 4 = 60

Hence, the product of the given numbers = 60

1. Given numbers are 5 and 7. We observe that 5 and 7 are co-prime numbers.

Hence, the HCF is 1.

1. We have 25 × 1 = 25; 25 × 2 = 50; 25 × 3 = 75

Hence, the required multiples are 25, 50 and 75.

1. (a) Possible factors of 12 are:

12 = 1 × 12; 12 = 2 × 6; 12 = 3 × 4

Hence, the factors of 12 are 1, 2, 3, 4, 6 and 12.

(b) Possible factors of 18 are:

18 = 1 × 18; 18 = 2 × 9; 18 = 3 × 6

Hence, the factors of 18 are 1, 2, 3, 6, 9 and 18.

1. First three multiples of 11 are:

11 x 1 = 11; 11 x 2 = 22; 11 x 3 = 33

Hence, the required multiples are: 11,22 and 33.

1. Pairs of twin prime numbers are: (3, 5), (5, 7), (11, 13), (17, 19).

Short Answer:

1. Given that: 32 + 96 ÷ (7 + 9)

= 32 + 96 ÷ 16 (Using BODMAS)

= 32 + 6 = 38

1. Given that: 18 + {1 + (5 – 3) × 5} (Using BODMAS)

= 18 + {1 + 2 × 5} = 18 + {1 + 10}

= 18 + 11 = 29.

1. Here 1,10,011 = 1,10,000 + 11

= 11 × 10,000 + 11 × 1

= 11 × (10,000 + 1)

= 11 × 10,001

It is clear that 11 is a factor of 11 × 10,001.

1. Hence, 11 is a factor of 1,10,011

The product of two numbers is 144.

∴ The possible factors are 1 × 144, 2 × 72, 3 × 48, 4 × 36, 6 × 24, 8 × 18, 9 × 16, 12 × 12

Here, we observe that out of these factors, we take 9 and 16.

Product = 9 × 16 = 144 and sum = 9 + 16 = 25

Hence, the required numbers are 9 and 16.

1. Sum of the digits at odd places = 6 + 1 + 8 = 15

Sum of the digits at even places = 3 + 0 = 3

Difference of the two sums = 15 – 3 = 12,

which is neither 0 nor the multiple of 11.

Hence, 80136 is not divisible by 11.

1. Given that: HCF = 6

LCM = 120

Let the two numbers be a and b, where a = 24, b = ?

We know that: a × b = HCF × LCM

⇒ 24 × b = 6 × 120

⇒ b = 6 × 12024

⇒ b = 30

Hence, the other number is 30.

1. Given numbers are 12 and 30

12 = 2 × 2 × 3;

30 = 2 × 3 × 5

∴ LCM = 2 × 2 × 3 × 5 = 60

Hence, the LCM of 12 and 30 = 60.

1. Given numbers are 18, 24 and 32, we have

Thus, LCM = 2 × 2 × 2 × 2 × 2 × 3 × 3 = 288

The smallest 4-digit number = 1000

Now, we write multiples of 288, till we get a 4-digit number.

288 × 1 = 288, 288 × 2 = 576,

288 × 3 = 864, 288 × 4 = 1152

Hence, 1152 is the required number.

1. Given numbers are 82 and 132 and the remainders are 1 and 6 respectively.

We have, 82 – 1 = 81 and 132 – 6 = 126

So, we need to find the HCF of 81 and 126

Common factor is 3 (occurring twice).

∴ HCF = 3 × 3 = 9

1. Hence, the required number is 9.

Given numbers are 455, 582 and 710 and the respective remainders are 14, 15 and 17.

We have 455 – 14 = 441, 582 – 15 = 567 and 710 – 17 = 693.

Now let us find their HCF.

Common factors are 3 and 7.

∴ HCF = 3 × 7 = 21

Hence, the required number is 21.

Long Answer:

1. Using BODMAS Rule, we have

40 + [20 – {28 ÷ 7 – 3 + (30 – 5 of 4)}]

= 40 + [20 – {28 ÷ 7 – 3 + (30 – 20)}]

= 40 + [20 – {28 ÷ 7 – 3 + 10}]

= 40 + [20 – [4 – 3 + 10}]

= 40 + [20-11] = 40 + 9 = 49.

1. To arrange the books in the required way,

we have to find the greatest number that divides 336, 192 and 144 exactly.

So, HCF of 336, 192 and 144 is

Common factors are 2 × 2 × 2 × 2 × 3 = 48

∴ HCF = 48, i.e., each stack contains 48 books.

∴ Number of stacks of English books = 336 + 48 = 7

Number of stacks of Hindi books = 192 4 – 48 = 4

Number of stacks of Urdu books = 144 4 – 48 = 3

1. We have

Number of cows = 111

Number of sheep = 185

Number of goats = 296

According to the condition of the boatsman, we need to find HCF of 111, 185 and 296

111 = 3 × 37;

185 = 5 × 37;

296 = 2 × 2 × 2 × 37

∴ the HCF = 37

So, the number of animals of same kind = 37.

Number of trips

=11137 +18537 +29637

= 3 + 5 + 8 = 16

Hence, the number of animals in each trip = 37

and the number of trips = 16

1. The number of participants in each room must be the HCF of 60, 96 and 144.

∴ 60 = 2 × 2 × 3 × 5

96 = 2 × 2 × 2 × 2 × 2 × 3

144 = 2 × 2 × 2 × 2 × 3 × 3

HCF = 2 × 2 × 3 = 12

Number of rooms required

= 6012 + 9612 + 14412

= 5 + 8 + 12 = 25

Hence, number of participants = 12

in each room and number of rooms required = 25.

Assertion and Reason Answers:

1) a) Both A and R are true and R is the correct explanation of A

2) a) Both A and R are true and R is the correct explanation of A

 

Download PDF Notes and Excercise solution of the Chapter.

 

Chapter 4: Basic Geometrical Ideas

 

Please Visit Readspot for Hindi Medium Study Material